What is the 10th term in the sequence 5 8 11 14
32
Therefore, the 10th term is 32.
What is the expression for the nth term of 5 8 11 14 17
The nth term is 3n+2. The given sequence is in A.P. =5+3n-3 =3n+2. THIS IS THE ANSWER.
What is the missing term in the sequence 2 5 8 11 14
Answer and Explanation: The next number in the list of numbers 2, 5, 8, 11, 14, . . . is 17. Notice that the difference between each consecutive term in this sequence is 3.
What is the number of terms 5 8 11 14 in the given ap series
Hence, in the given sequence, the number of terms in 5, 8, 11,14, 17,__, 50 are 16.
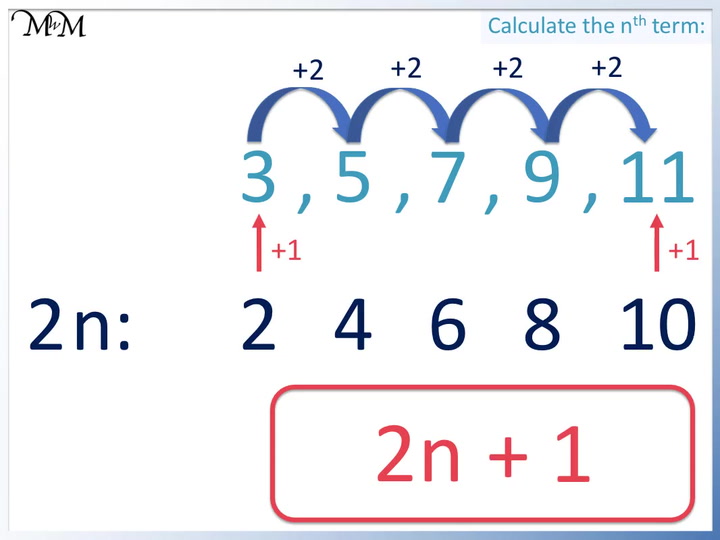
What is the nth term rule of 2 5 8 11
an=3n−1.
What is the nth term of 9 11 13 15 17
Solution: Given, the arithmetic sequence is 9, 11, 13, 15,…. We have to find the equation for the nth term of the sequence. Therefore, the equation for the nth term of the sequence is an = 2n + 7.
What is the 30 term of the sequence 5 8 11 14
Hence, the 30th term is equal to 92.
What is the predicted next term of the sequence 8 11 14 17
This shows that to get to the next term of the sequence, you add 3. Therefore the next term of the sequence is 20.
What is the next three terms of 2 5 8 11 14
2, 5, 8, 11, 14, 17, 20, 23, 26, 29, 32, ..
What is the 10th term in the sequence 2 5 8 11
29
2,5,8,11,…. 10th term of the sequence is 29.
Is 5 8 11 14 an AP if so then what will be the 100 term
The correct Answer is (i) Is an A.P. (ii). t100=302. (iii) 92 is in the given A.P. , (iv) 61 is not in the given A.P.
How many terms are there in the arithmetic series below 5 8 11 14 17 20 50
16
5, 8, 11, 14, 17, 20……… 50. Explanation: nth term = first_term + (number_of_terms – 1)common_differnce., 50 = 5 + (n-1)3, n=16.
What is the sequence of 5 8 11 14
Sequence A: 5 , 8 , 11 , 14 , 17 , … For sequence A, if we add 3 to the first number we will get the second number. This works for any pair of consecutive numbers. The second number plus 3 is the third number: 8 + 3 = 11, and so on.
How do you find the 10th term of a sequence
Solution: To find a specific term of an arithmetic sequence, we use the formula for finding the nth term. Step 1: The nth term of an arithmetic sequence is given by an = a + (n – 1)d. So, to find the nth term, substitute the given values a = 2 and d = 3 into the formula.
What is the n rule for this sequence 9 11 13 15 17 19
Solution: Given, the arithmetic sequence is 9, 11, 13, 15,…. We have to find the equation for the nth term of the sequence. Therefore, the equation for the nth term of the sequence is an = 2n + 7.
What is the nth term of 7 11 15 19 23
4n + 3
The original sequence we wanted: 7, 11, 15, 19, 23, … To get the terms in the original sequence, we need to add on 3 to each of the terms in the 4n sequence. Hence, the general formula for the nth term of the original sequence is 4n + 3.
What is the 20th term of 5 8 11 14
So, the 20th term is 59. $\therefore $ The 20th term of the given sequence 2, 5, 8, 11, 14, 17…… is 59, and the sum of 20 terms of the sequence 2, 5, 8, 11, 14, 17…… is 610.
What type of sequence is 5 8 11 14 17
arithmetic sequences
Sequences of numbers that follow a pattern of adding a fixed number from one term to the next are called arithmetic sequences. The following sequences are arithmetic sequences: Sequence A: 5 , 8 , 11 , 14 , 17 , …
What’s the nth term of 5 8 11 14
The nth term of an A.P. 5, 8, 11, 14, …… is 68.
Is 5151 a term of the AP 5 8 11 14 5 8 11 14
Text Solution
Here a = 5 and d =(8-5) = 3. ⇒3n=49⇒n=1612. But, the number of terms cannot be a fraction. ∴ 51 is not a term of the given AP.
What is the sum of the first 10 terms of the AP 5 8 11 14
Sum of first 10 terms S10=n2(2a+(n−1)d)=102(2×5+(10−1)3)=185.
What is the 10th term of the arithmetic sequence 2 5 8 11
29
2,5,8,11,…. 10th term of the sequence is 29.
What is the arithmetic series of 2 5 8 11 14 17 20
Arithmetic sequences
2,5,8,11,14,17,20,23,26,29,32…
What is the common difference of 5 8 11 14
3
The common difference of the sequence 5,8,11,14 is 3.
What is the recurrence relation for the sequence s 5 8 11 14 17
Sequence = 5,8,11,14,17,… Therefore, From all the above observation it can be seen that each Term has a recurrence relation of sum of 3.