What is the general term of 1 2 4 8 16
It is a geometric sequence.
What is the common ratio of 1 2 4 8 16
This sequence has a common ratio ( 2 ), rather than a common difference. It is a geometric sequence, not an arithmetic one.
What is the next term in the sequence 2 4 8 16 ____
The sequence then continues: 2,4,8,16,32,64,128,256,512,1024,2048,…
What is the general term of 4 8 16
Summary: The nth term of the geometric sequence 4, 8, 16, 32, … is an = 4(2)n – 1.
What is the nth term for 1 2 4 8 16 32
So, the th term rule for the given sequence is: a n = 2 n − 1 , n ∈ [ 1 , ∞ ) .
What is the general term for 1 1 2 3 5 8
Fibonacci Numbers (Sequence):
1,1,2,3,5,8,13,21,34,55,89,144,233,377,… Fn=Fn−2+Fn−1 where n≥2 . Each term of the sequence , after the first two, is the sum of the two previous terms. This sequence of numbers was first created by Leonardo Fibonacci in 1202 .
Is the following a geometric series 1 2 4 8 16
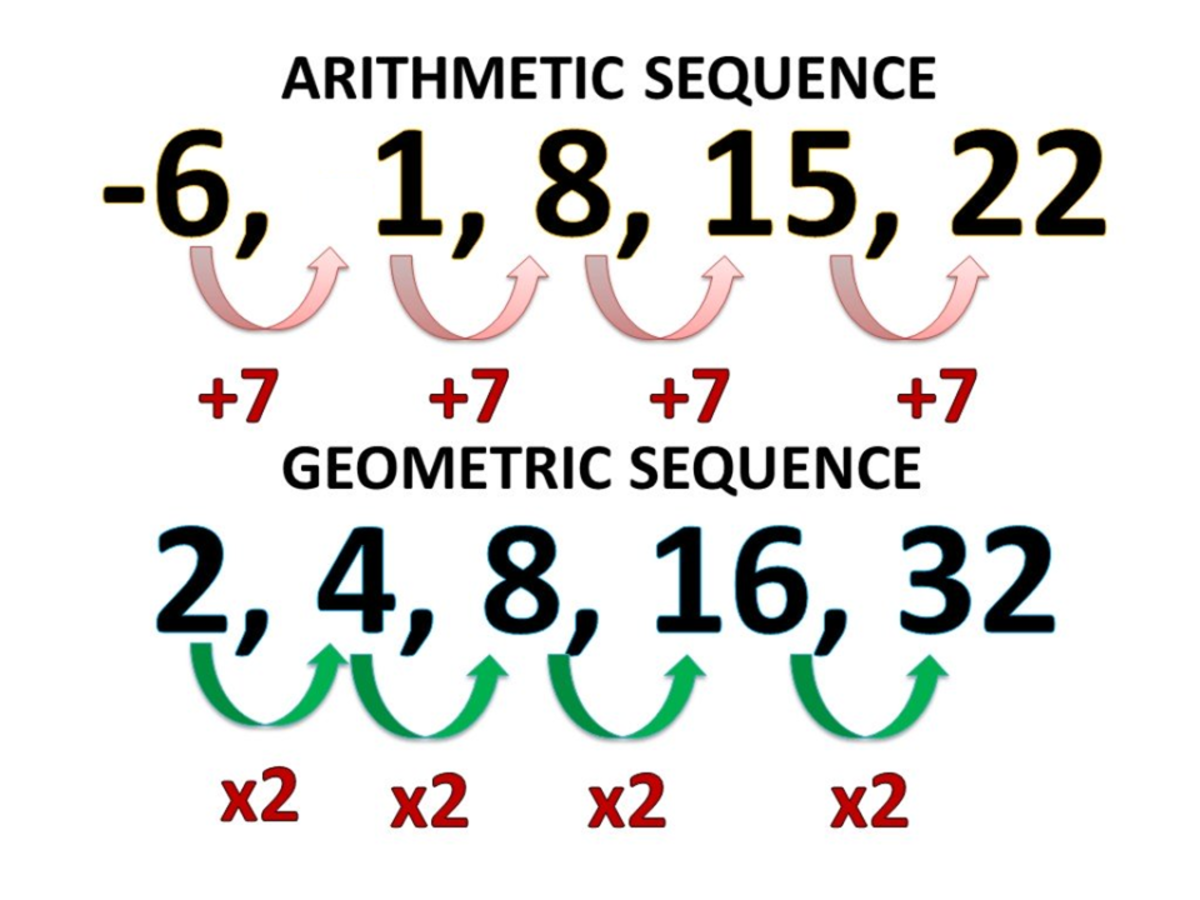
The sequence 1,2,4,8,16,… is a geometric sequence with common ratio 2, since each term is obtained from the preceding one by doubling. The sequence 9,3,1,1/3,… is a geometric sequence with common ratio 1/3.
What is the common ratio for the sequence 1 4 16 64 4 2
4
The common ratio of the geometric sequence $ 1,4,16,64,….. $ is 4. So, the correct answer is “4”.
What is the next number in the sequence 1 2 4 8 16 31
, giving the sequence 1, 2, 4, 8, 16, 31, 57, 99, 163, 256, …
Which of the following is an arithmetic sequence 1 2 4 8 16
Expert-Verified Answer
The first sequence is 2, 4, 8, 16, … Since the differences of the terms are not equal, then the sequence is not an arithmetic sequence.
What is the general term for the sequence 2 4 8 16 32 n 2 2 n 2n
Solution: The given sequence is 2, 4, 8, 16, 32, …. Therefore, the general term is an = 2n.
What is the general term of the sequence 1 2 4 8
Any term can be calculated by using the formula: tn = a rn- 1. So in the given question i.e. 1, 2, 4, 8 the next term is obtained on multiplying 8 by 2 . So the answer is 16.
What is the nth term of 1 ⁄ 2 1 ⁄ 4 1 8 1 16
Answer: It is 1/32, because each ane is multipllied by 1/2 to give next no. the we will multiply 1/16 by 2 that will give 1/32 as an answer.
What is the general term of 1 2 2 3 3 4
For the given sequence the general term could be defined as n/(n + 1), where 'n' is clearly a natural number. So starting with the number 1/2 (with starting n = 1) the sequence goes on as follows : 1/2, 2/3, 3/4, 4/5, 5/6, 6/7…. So the very next number to 4/5 is 5/6.
Is 1 2 4 8 16 infinite or finite
In mathematics, 1 + 2 + 4 + 8 + ⋯ is the infinite series whose terms are the successive powers of two. As a geometric series, it is characterized by its first term, 1, and its common ratio, 2. As a series of real numbers it diverges to infinity, so the sum of this series is infinity.
Is 2 4 8 16 32 a geometric sequence
Answer: The 2 + 4 + 8 + 16 + 32 + . . . series is a geometric sequence or a geometric progression (G.P.'s).
What is the general term of the sequence 1 4 16 64
By multiplication rule you will get the answer. Multiply each of the number by number 4. The sequence for 1,4,16,64 is 1*4 =4 , 4*4=16, 16*4= 64, 64*4 = 256.
What is the common ratio of the geometric sequence 2 4 8 16 32 64
2
An example of a Geometric sequence is 2, 4, 8, 16, 32, 64, …, where the common ratio is 2.
What is the sequence of 1 2 4 8 15
The values of Cn for n = 0, 1, 2, … are given by 1, 2, 4, 8, 15, 26, 42, 64, 93, 130, 176, 232, … (sequence A000125 in the OEIS).
What is the next number in the sequence 1 4 2 8 6 24 22 ________
Text Solution
The missing number is 88−2=86.
How do you find the general term of a sequence
The general term for a sequence follows a certain pattern. The successive terms are getting by adding or multiplying a number to the previous term. Sometimes each term of the series follows an expression. The general term of an AP is T n = a + ( n – 1 ) d .
What do we call this sequence 1 1 2 3 5 8 *
And this type of sequence is called a Fibonacci sequence. Hence, the answer is Fibonacci sequence.
How do you find the general term
Within on the bottom. And if you look at the top this is always one less than n. Minus 1 because 1 minus 1 is 0 2 minus 1 is 1 3 minus 1 is 2 4 minus 1 is 3 5 minus 1 is 4 6 minus 1 is 5.
What is the nth term of 1 1 2 3 5 8
1,1,2,3,5,8,13,21,34,55,89,144,233,377,… Fn=Fn−2+Fn−1 where n≥2 . Each term of the sequence , after the first two, is the sum of the two previous terms. This sequence of numbers was first created by Leonardo Fibonacci in 1202 .
Is 1 2 3 4 5 6 finite or infinite
finite
A set that has a finite number of elements is said to be a finite set, for example, set D = {1, 2, 3, 4, 5, 6} is a finite set with 6 elements. If a set is not finite, then it is an infinite set, for example, a set of all points in a plane is an infinite set as there is no limit in the set.