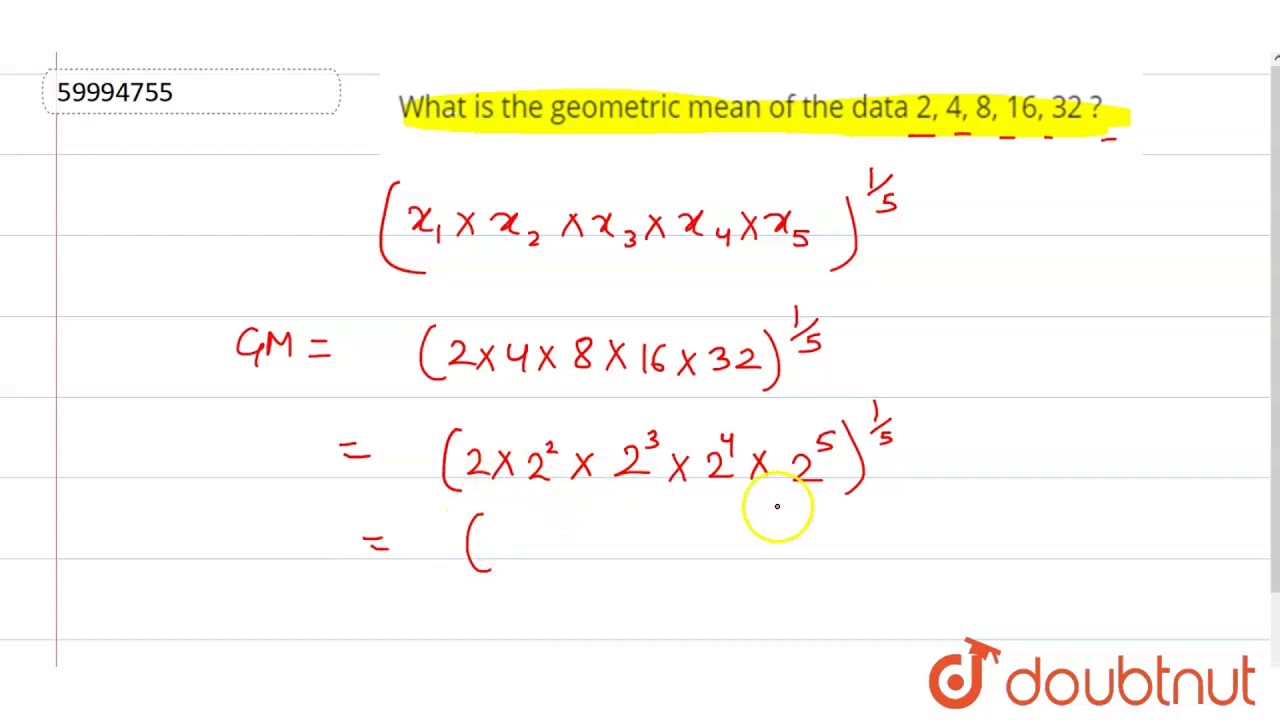
What is the general term of 4 8 16 32 64
Summary: The nth term of the geometric sequence 4, 8, 16, 32, … is an = 4(2)n – 1.
Is 4 8 16 32 64 a geometric sequence
This is a geometric sequence since there is a common ratio between each term. In this case, multiplying the previous term in the sequence by 2 gives the next term.
What is the next term in the geometric sequence 2 4 8 16 32
1, 2, 4, 8, 16, 32, 64, 128, 256, … This sequence has a factor of 2 between each number. Each term (except the first term) is found by multiplying the previous term by 2.
What is the common ratio of 4 8 16 32 64
To find the common ratio of a geometric sequence you would divide each term by the term before it. The common ratio is one-fourth (1/4). The first five terms are 4, -8, 16, -32, and 64.
What is the next term of the sequence 2 4 8 16 32 64
To determine the next number in the sequence, we can see that each number is double the previous one. So the next number in the sequence is 128. Therefore, the complete sequence is: 2, 4, 8, 16, 32, 64, 128.
Which is the wrong term in sequence 1 2 4 8 16 32 64 96
Each term is double the preceding term so 96 is the wrong term It should be 128.
Is 2 4 8 16 32 a geometric sequence
Answer: The 2 + 4 + 8 + 16 + 32 + . . . series is a geometric sequence or a geometric progression (G.P.'s).
What is the common ratio of the geometric sequence below 2 4 8 16 32 4 2 2 4 2 4 4 2
Given, the geometric sequence is -2, 4, -8, 16, -32,…. We have to find the common ratio of the given geometric sequence. r = b/a = c/b = d/c. Therefore, the common ratio is r = -2.
What is the common ratio of the geometric sequence 2 4 8 16 32
2
Given the geometric sequence 2,4,8,16,… . To find the common ratio , find the ratio between a term and the term preceding it. 2 is the common ratio.
What is the common ratio for 1 2 4 8 16 32
-2 is the common ratio.
What is the general or nth term of the sequence 2 4 8 16 32
Solution: The given sequence is 2, 4, 8, 16, 32, …. Therefore, the general term is an = 2n.
What are the next three terms in the sequence 2 4 8 16 32 64 __ __ __
The sequence then continues: 2,4,8,16,32,64,128,256,512,1024,2048,…
What is the common ratio in the geometric sequence 2 4 8 16 32
2
Example: Given the geometric sequence 2,4,8,16,… . To find the common ratio , find the ratio between a term and the term preceding it. 2 is the common ratio.
What is the nth term of 2 4 8 16 32
So, the next term is: 2 × 2 × 2 × 2 × 2 × 2 = 64.
Is this a geometric sequence 2 4 8 16 32
Answer: The 2 + 4 + 8 + 16 + 32 + . . . series is a geometric sequence or a geometric progression (G.P.'s).
What is the common ratio for the sequence 1 4 16 64 4 2
4
The common ratio of the geometric sequence $ 1,4,16,64,….. $ is 4. So, the correct answer is “4”.
What are the next two terms in the sequence 4 8 16 32 64
To determine the next number in the sequence, we can see that each number is double the previous one. So the next number in the sequence is 128. Therefore, the complete sequence is: 2, 4, 8, 16, 32, 64, 128.
What is the 20th term of the progression 4 8 16 32
Solution: If the ratio between two consecutive terms in a sequence is same throughout, then the sequence is called a geometric progression. Thus, the 20th term of the sequence is 2097152.
What is the next term in the sequence 2 4 8 16 32 64
The sequence then continues: 2,4,8,16,32,64,128,256,512,1024,2048,…
Is 2 4 6 8 16 a geometric sequence
It is a geometric sequence.
What is the common ratio between successive terms in the sequence 2 4 16 32 64
In the present question we have -4/2 = 8/-4 =-16/8 =32/16 = -64/32 = -2. Hence the common ratio between successive terms of the given sequence = -2.
Is 2 4 8 16 32 an arithmetic sequence
Answer: The 2 + 4 + 8 + 16 + 32 + . . . series is a geometric sequence or a geometric progression (G.P.'s).
What is the 20th term of the series 2 4 4 6 6 8 nth term
=40(42)=1680.
What is the nth term for 2 4 6 8 16 32
In this situation the pattern is x2 (aka doubling) each time. Therefore the nth term would be 2^n (meaning 2 to the power of n). We can check this by imputing numbers in place of n: 2^1 = 2 2^2 = 4 2^3 = 8 2^4 = 16 You won't get there with the nth-difference approach.
What is the common ratio of the sequence 2 4 8 16 32
Given, the geometric sequence is -2, 4, -8, 16, -32,…. We have to find the common ratio of the given geometric sequence. r = b/a = c/b = d/c. Therefore, the common ratio is r = -2.