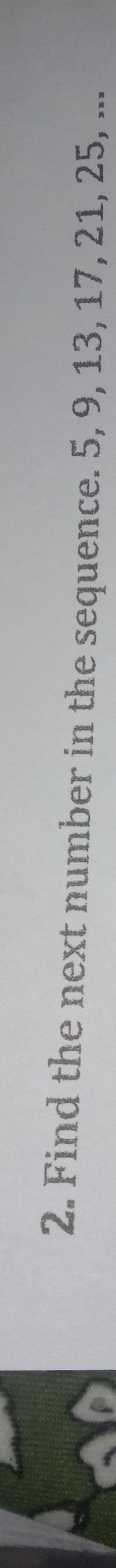Which number comes next in the sequence 5 9 13 17 21
1, 5, 9, 13, 17, 21, 25, 29, . . ., 4n+1, . . . In general, the terms of an arithmetic sequence with the first term a0 and common difference d, have the form an = dn+a0 (n=0,1,2,…).
What type of series is listed below 1 5 9 13 17
This is an arithmetic sequence since there is a common difference between each term. In this case, adding 4 to the previous term in the sequence gives the next term. In other words, an=a1+d(n−1) a n = a 1 + d ( n – 1 ) . This is the formula of an arithmetic sequence.
What is the common difference of the sequence 5 9 13 17 21
{1,5,9,13,17,21,25,…} is an arithmetic sequence with common difference of 4 .
What is the common difference of 5 9 13 17
4
And, we know that the difference between the consecutive numbers is 4. Hence, we have found the common difference of the arithmetic sequence 5, 9, 13, 17,…. The common difference is 4.
What is the rule of pattern 5 9 13 17
Answer and Explanation:
The sequence is 5 , 9 , 13 , 17 , ⋯ . As the difference between consecutive terms is equal, the sequence is an arithmetic progression. The first term of the AP is 5 and the common difference is 4. So, the general term of the AP is a n = 5 + 4 ( n − 1 ) .
What is the sum of the first 30 terms of 5 9 13 17
The obtained sum is 1890.
What is common difference in the sequence 5 9 13 17 21
{1,5,9,13,17,21,25,…} is an arithmetic sequence with common difference of 4 .
What are the number patterns of 3 5 8 13 21
Fibonacci Numbers (Sequence):
1,1,2,3,5,8,13,21,34,55,89,144,233,377,… Fn=Fn−2+Fn−1 where n≥2 . Each term of the sequence , after the first two, is the sum of the two previous terms. This sequence of numbers was first created by Leonardo Fibonacci in 1202 .
What is the sum of 23 terms of the sequence 5 9 13 17 is k
Now, Sn=n2[2a+(n−1)d]∴S23=232[2(5)+(23−1)4] =232×98 =1127.
What is the sum of the first 23 terms of the AP 5 9 13 17
Hence, the sum of 23 terms of the given AP is 1127.
What is the 5 8 13 21 34 sequence
The Fibonacci sequence
The Fibonacci sequence is a series of numbers where a number is the addition of the last two numbers, starting with 0, and 1. The Fibonacci Sequence: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55… This guide provides you with a framework for how to transition your team to agile.
What is the rule for the sequence 3 5 8 13 21 34
Fibonacci Numbers (Sequence):
1,1,2,3,5,8,13,21,34,55,89,144,233,377,… Fn=Fn−2+Fn−1 where n≥2 . Each term of the sequence , after the first two, is the sum of the two previous terms. This sequence of numbers was first created by Leonardo Fibonacci in 1202 .
What are the next three terms of the arithmetic sequence 9 13 17 21 25
9,13,17,21,25,29,33,37,41,45,49…
What is the sum of the first n terms of 1 5 9 13 17
Answer: Sum of arithmetic sequence 1, 5, 9, 13, …… (up to 20 terms) = 780.
What type of sequence is 5 8 13 21 34 35
Fibonacci Numbers (Sequence):
1,1,2,3,5,8,13,21,34,55,89,144,233,377,…
What is the pattern for 3 5 8 13 21
The Fibonacci sequence is a famous group of numbers beginning with 0 and 1 in which each number is the sum of the two before it. It begins 0, 1, 1, 2, 3, 5, 8, 13, 21 and continues infinitely.
What is the common difference of the arithmetic sequence 5 9 13 17 21
4
Example 1:
{1,5,9,13,17,21,25,…} is an arithmetic sequence with common difference of 4 .
What are the next three terms of the arithmetic sequence 5 9 13 17
5,9,13,17,21,25,29,33,37.
Is 1 5 9 13 17 an arithmetic sequence
An arithmetic sequence (also known as an arithmetic progression) is a sequence of numbers in which the difference between consecutive terms is always the same. For example, in the arithmetic sequence 1, 5, 9, 13, 17, …, the difference is always 4. This is called the common difference.
What is the sequence 5 8 13 21 34
The Fibonacci sequence
The sequence follows the rule that each number is equal to the sum of the preceding two numbers. The Fibonacci sequence begins with the following 14 integers: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233 … Each number, starting with the third, adheres to the prescribed formula.
What is the sequence 1 2 3 4 5 8 13 21 34
The Fibonacci sequence
The Fibonacci sequence begins with the following 14 integers: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233 … Each number, starting with the third, adheres to the prescribed formula. For example, the seventh number, 8, is preceded by 3 and 5, which add up to 8.
What is the rule of 1 1 2 3 5 8 13 21
The Fibonacci sequence is the series of numbers: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34… In this series, the next number is found by adding the two numbers before it. Hence, the next term in the series is 8 + 13 = 21. Q.
Which of the following is the common difference in the arithmetic sequence 5 9 13 17
4
For example, in the arithmetic sequence 1, 5, 9, 13, 17, …, the difference is always 4. This is called the common difference. If the first term of the sequence is a and the common difference is d, then the arithmetic sequence can be written as a, a+d, a+2d, a+3d, …, a+(n−1)d, …
What is the pattern rule for 5 9 13 17
This is an arithmetic sequence since there is a common difference between each term. In this case, adding 4 to the previous term in the sequence gives the next term.
What is the pattern of 1 1 2 3 5 8 13 21 34 55
The Fibonacci sequence
The Fibonacci sequence is the series of numbers where each number is the sum of the two preceding numbers. For example, 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, …